Parabola with Circular Directrix

Erin Mueller

Construct the locus of points equidistant from a fixed point F and a circle. In other words, repeat the parabola construction but use a circle as the “directrix”. Let F be any point in the plane other than the center of the circle. Assume F is not on the circle; it can be either inside or outside.

Below is a picture of what occurs when we trace the locus of points equidistant from a fixed point (F) and a circle.

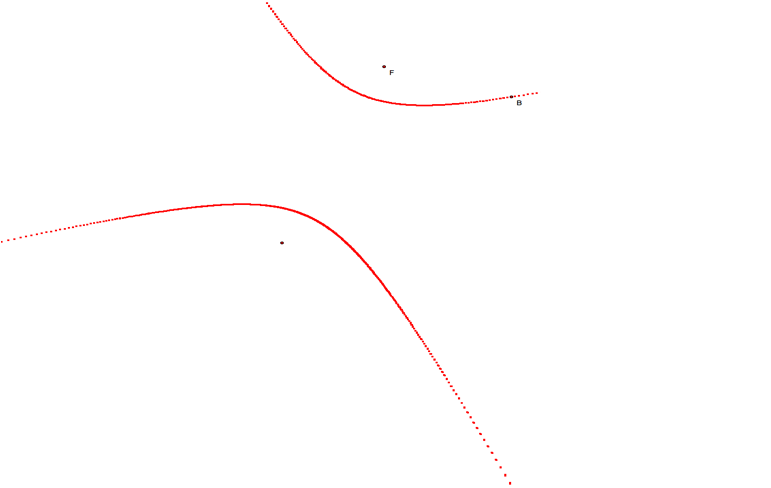
As we can see from above, we have a hyperbola when our focus point (F) is outside of the circle. Below, we can see all of the hidden parts of our picture to get a good look at what is REALLY happening.

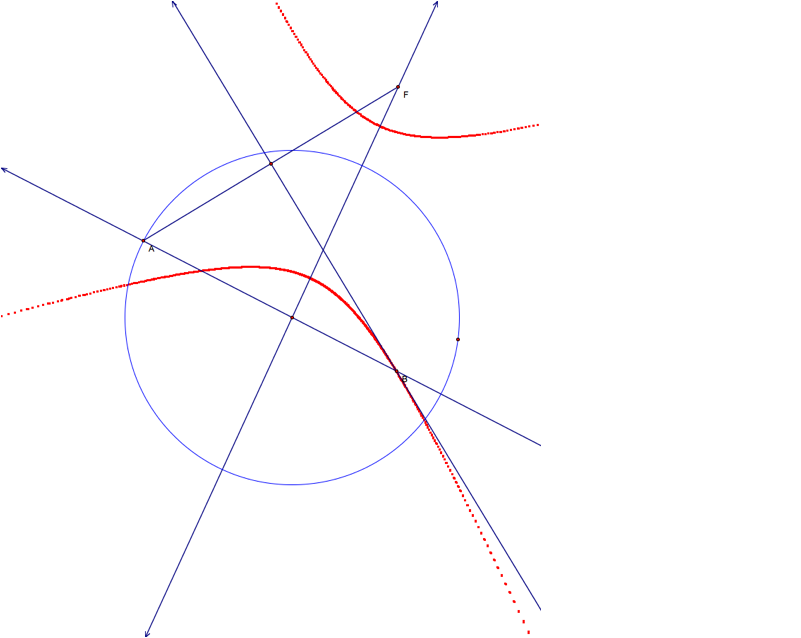
Our intersection point (B) is being traced to form the hyperbola as point (A) goes around our circle. When this happens, the two lines that intersect to form (B) are also being moved. When these two lines become parallel, we have our asymptotes. Only as long as these two line intersect, will we have a point to trace that will become a hyperbola. Notice that our focus (F) and the center of the circle are the two foci points of the hyperbola.

Now look what happens when we trace the midpoint of segment AF. What shape do you think we will see?

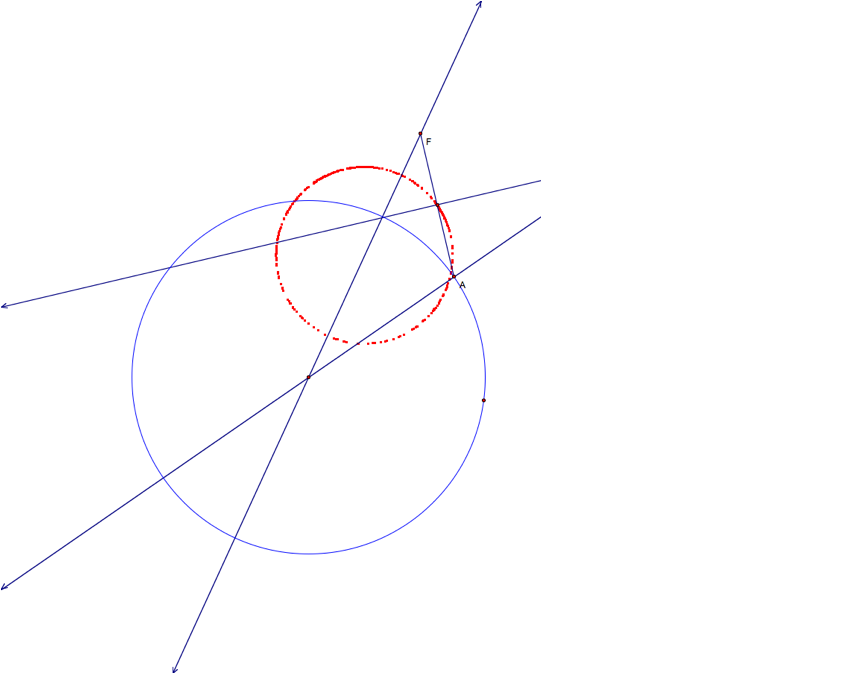
If you guessed a circle, you would be correct. The center of this new circle is also the center of our hyperbola.

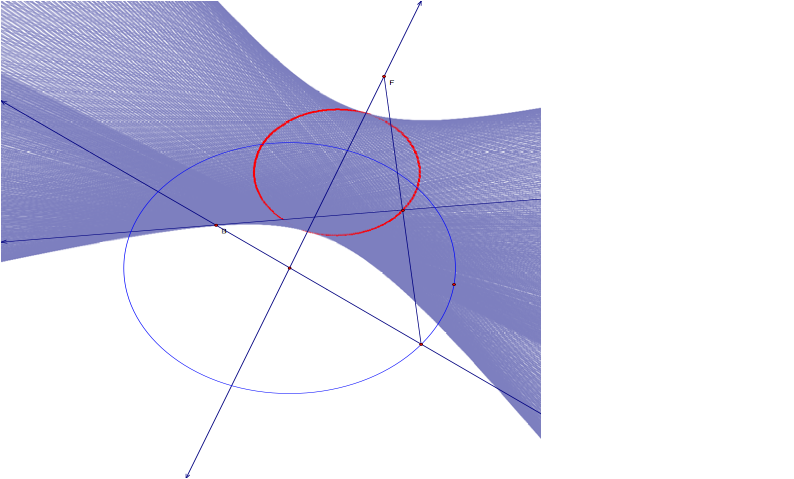
Now what if the focus (F) were inside the circle.

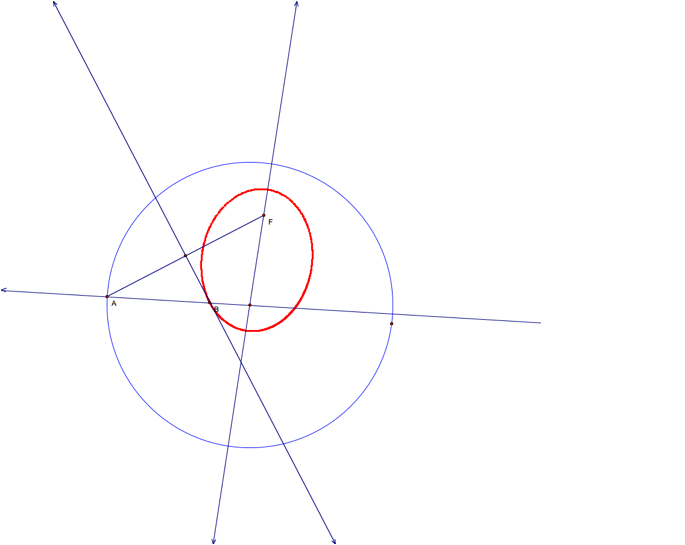
We now see an ellipse. The foci points of the ellipse are the same as for the hyperbola; (F) and the center of the circle. Using what we know about ellipses (the fact that the total distance from F(1) to F(2) to B is constant), we can see how the intersection point (B) would remain inside the circle.

Again, we can also make a circle similar to the circle formed inside of the hyperbola. Only this time, the circle will be tangent to the outside of the ellipse.

Pretty neat huh?

Go Home